by Ken Ellis Autoharp players have a bad reputation among other musicians for always being out of tune. The appearance on the market of inexpensive chromatic tuners made it much easier to keep an autoharp in tune. So we no longer have an excuse. While it is true that 36 or 37 strings take longer to tune than the 4 to 8 strings on most string instruments, it is also true that if you keep your autoharp in tune, it will tend to stay in tune. This will significantly reduce the amount of time you have to spend tuning and also help you to stay in tune during a long jam or performance.   You may be surprised to find out that, no matter how accurate a tuner is, it takes more than a tuner to really get an autoharp in tune. To help you understand why this is so, the first part of this article will describe how strings vibrate. If you are in a hurry to get tuned, you may skip the first part and go directly to the second, where I give two step-by-step methods for accurate tuning.   Strings   When a string is under tension and is pulled to one side, the tension pulls the string back toward its at-rest position. The mass of the string gives it momentum, however, so it does not simply return to rest but overshoots. At this point the tension acts on it to reverse its direction, then the process repeats, causing the string to vibrate. The higher the string tension, the stronger the restoring force, and the faster the string attempts to return to the center of its motion. Thus a higher tension produces a higher frequency sound. When plucked, a string vibrates at multiple frequencies simultaneously. The fundamental frequency has a wavelength that is twice the length of the string. This frequency usually determines the pitch of the note. The higher frequencies are called partials. The partials have shorter wavelengths than the fundamental. There are an exact number of half wavelengths of the partials between the ends of the string. When the frequency of a partial is an integer multiple of the fundamental, it is called a harmonic of that frequency. 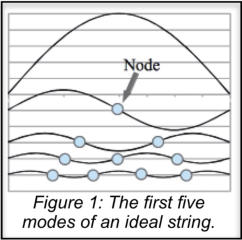Figure 1 shows how the string would bend if it could vibrate at only one frequency at a time. The harmonic and the first four partials are shown. For every frequency there are locations on the string where the vibration is zero for that frequency. These points are called the nodes of that frequency and are represented as dots in Figure 1. The nodes are separated by one-half of the wavelength of the frequency. Every frequency has a node at each end of the string.   There is a YouTube video that is a great illustration of how strings vibrate: <div class="player-unavailable"><h1 class="message">An error occurred.</h1><div class="submessage"><a href="http://www.youtube.com/watch?v=8B6jOUzBKYc" target="_blank">Try watching this video on www.youtube.com</a>, or enable JavaScript if it is disabled in your browser.</div></div> In this video you can see the partials of the guitar strings while it is being played.   You probably already know that a video only produces the illusion of movement. It does this by presenting a series of still pictures ('frames') in rapid succession, so rapid that your brain can't keep up. You  perceive motion when an object changes position in a series of frames.  Videos are recorded by  taking a series of frames in rapid succession. If the frame rate is high compared to the speed of moving objects, they will appear to be frozen in each frame. The effect is equivalent to 'freezing' motion using a strobe light.   In the video the strings sometimes have a partial at a frequency that is close to a multiple of the frame rate. The strings then appear to vibrate at a frequency equal to the difference between the partial's frequency and the closest multiple of the frame rate. When this difference is zero or very small, the strings appear to be stationary or slowly moving. You typically only see one partial/mode at a time, while the string is actually vibrating in many modes simultaneously. Ideal Strings   An ideal string is perfectly flexible. Its fixed ends can also pivot freely. The frequencies of the partials of an ideal string are exact multiples of the fundamental frequency, making them harmonics. You can hear a partial if you touch a vibrating string at one of the nodes. This damps out any frequency that does not have a node at that point. Try plucking a string rapidly as you lightly run a finger from your other hand along the string. You will hear a range of tones, with the partials growing significantly louder as you approach a node.   Non-Ideal Strings   Real strings are not perfectly flexible. They have a stiffness that increases with frequency. Higher frequencies encounter more stiffness than lower frequencies because they require bending the string to a tighter curve. The stiffness increases the restoring force on the string so that it moves back towards its at-rest position faster than it would have due to string tension alone. This produces “inharmonicity” which shifts the frequencies of the partials slightly higher than those of the true harmonics, each partial by a different amount.   It is usually desirable to have similar string length and tension for all strings on an instrument. The only way to achieve this is to increase the mass density of the strings in order to get the lower notes. If you simply increased the diameter of the string to increase the mass density for a bass note, the lowest strings would be so stiff that the inharmonicity would be annoying. It would also become difficult to pluck, requiring much more force than the lighter strings and making the instrument harder to play. For this reason, we use wound strings for the bass notes. The winding increases the mass density significantly without a significant increase in stiffness.   Tuning   Autoharp strings are relatively stiff due to their short length and relatively large diameter (compared to guitar strings, anyway). Thus inharmonicity is more apparent in autoharps than other instruments. This can cause problems when tuning, and is probably a factor in why Bryan Bowers developed his tuning method to be what it is.   Bryan starts with a rough tuning with a chromatic tuner. He begins with the lowest note and tunes the same note in all the other octaves before proceeding to the next note. By tuning strings that are spaced far apart, the stresses on the harp body stay reasonably balanced throughout the tuning process. This not only prevents a possible catastrophic structural failure (which would be bad), but also makes it easier to keep the harp in tune later. There may be around 1800 to 2000 pounds of tension in the strings of an autoharp, so you can imagine that tuning all the strings on one side of the harp first could put a lot of stress on the box.   When he reaches the end of the first octave, Bryan isn't done with the rough tuning. He continues up the scale so that the strings in the second octave get tuned twice and those in the third, three times.   After the rough tuning, Bryan slowly plays arpeggios of each chord, listening for individual strings that don't sound right within the chord. When he finds a note that doesn't sound right, he plays a different chord containing the same note to ensure that a) he has the right string and 2) retuning doesn't throw off its sound when played in other chords. Sometimes a string sounds out of tune because the string next to it is out of tune. Listening to two chords that contain the presumed out-of-tune string will catch this. Finally, he plays a song or two to make sure that each note sounds correct in the musical context in which it will be played.   Now a chromatic tuner will tune the fundamental of each string. However, when tuning two strings separated by an octave, you are really matching the fundamental of the higher one to the first partial of the other. If the lower string has noticeable inharmonicity, the higher string will need to be raised in pitch slightly to match it, making it sharp relative to the chromatic tuner. I believe that this is one of the things that Bryan is correcting when playing chords/tunes and listening for out-of-tune strings.   I really like Bryan's tuning method, but unless I am changing strings I usually modify it as follows. Because I am often impatient, I usually don't want to tune strings three and four times with the tuner. After the first time through all the strings during rough tuning, I will go back to the strings in the octave in the middle of the harp (these would be the doubled strings on a diatonic harp) and tune them again with the tuner to make sure that the changing stresses during the rough tuning did not pull them out of tune. This gives one in-tune string at every note in the scale. 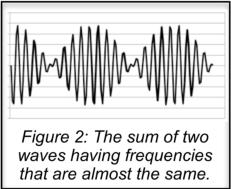Now when two notes are not quite in tune, the sound waves will add together as shown in Figure 2. We hear the note (the high frequency in the figure) modulated by a “beat frequency” (the low frequency envelope in the figure), so that it has kind of a “wah-ooh-wah-ooh” sound. The beat frequency is equal to the difference in the two frequencies. As the two notes are brought into tune, the beats slow down. They go away when the frequencies are exactly the same.   When tuning a diatonic harp I tune the second string in each unison pair to the first by adjusting it until the beating between notes is inaudible (to me, anyway). For both diatonic and chromatic harps I then walk up the scale, tuning the same note in all other octaves to the one in the middle octave before proceeding to the next note. If you have a quiet room, you can hear the beat frequency between the fundamental of one string and the first partial of another that is tuned an octave lower. You can tune the string that is not in the middle octave to eliminate the beat frequency. I find it helps to pluck the lower string first since the fundamental tends to die away faster than the first partial. Strings above the middle octave may need to be tuned a little sharp relative to the tuner in order to compensate for inharmonicity, and those below the middle octave a little flat.  After tuning all strings to the middle octave, I go through the chords and play a tune per Bryan's method and only occasionally need to do additional fine tuning.   I only use beat frequencies to tune octave intervals. You can tune other intervals with this method, but that only works well for a single key harp. This is because a perfect interval in one key is not an exact musical interval in all other keys (hence the need for the even-tempered scale). Tuning to exact intervals will also put you out of tune with respect to fretted instruments, which assume even temperament.   It is common for people to have trouble tuning the bass strings, even with a chromatic tuner. There are various reasons for this, which I won't go into here. Warren Fisher recommends that if you have trouble tuning the bass strings, touch the center of the string when plucking it to suppress the fundamental and get the first partial, then tune the partial using the tuner. An alternative trick is to pluck the string near its end. This causes the partials to be stronger and the fundamental to be weaker, but can make it easier for the tuner to determine the vibration frequency.   Postscript   So there you have it, the why and how of getting your autoharp in tune. I encourage you to follow one of these tuning methods before you play your autoharp. Then nobody can complain that you are out of tune. I also encourage you to tune after you are done playing for the day. If your autoharp is in tune when you put it away, it will be much less work to tune it the next time you want to play!   © Ken Ellis, 2015. All rights reserved. Return to Resources Page

How to Accurately Tune Your Autoharp